I am 'Huang,Yen-Chieh' at Taiwan. I am going to describe a perfect theory for structure of everything in the cosmos. This theory is an 'united philosophy' and is so perfect as to be able to explain everything in the cosmos. I call this 'united philosophy' 'squares theory'. There are important statements and figures in squares theory. Basically,the squares theory is to describe 'the pair phenomenon' in the cosmos. The two compositions of a 'pair' must be complementary to each other. Furthermore, the two statuses(rankings) of the two compositions of a 'pair' must be equal to each other! I declare again that the two compositions of a 'pair' must be complementary to each other, and the two statuses(rankings) of the two compositions of a 'pair' must be equal to each other! There are surely four sides in a square,and the area of any square must be 1, supposing we let the relative length of any side be 1. In all squares I use only 2 kinds of small rectangles to represent a being's dharma(way) at any time interval specified and at any site(volume) interval specified. We can say that there are only 2 kinds of dharmas(ways) in all squares. The 2 kinds of small rectangles or dharmas(ways) are black rectangles and white rectangles. The 2 kinds of dharmas(ways) are surely complementary to each other. There are only 2 'kinds' of small rectangles in any square, but there may be 'many' black rectangles and white rectangles in a square. The total area of all black rectangles in a square must be 1/2,and the total area of all white rectangles in a square must be 1/2,too. The value of time mentioned above is in the range of 0(,i.e. the beginning of the period of a being,) and 1(,i.e. the end of the period of a being), and the value of site(volume) mentioned above is in the range of 0(,i.e. the beginning of the height of a being,) and 1(,i.e. the end of the height of a being). We can indicate the time and the site(volume) of any point in a square by the Cartesian coordinate system. I declare that the lower-left corner of any square must be at the (0,0) point in the Cartesian coordinate system, the lower side of any square must be located between the (0,0) point and the (1,0) point of the positive X-axis in the Cartesian coordinate system, and the left side of any square must be located between the (0,0) point and the (0,1) point of the positive Y-axis in the Cartesian coordinate system. I declare that the positive X-axis in the Cartesian coordinate system is defined as time,and the positive Y-axis in the Cartesian coordinate system is defined as site(volume). The followings describe the time and site(volume) of the 4 sides of a square. 1.Left side from the (0,0) point to the (0,1) point: The time of left side is 0(,i.e. the beginning of the period of a being),and the site(volume) of left side is in the interval between 0(,i.e. the beginning of the height of a being,)and 1 (,i.e. the end of the height of a being). 2.Right side from the (1,0) point to the (1,1) point: The time of right side is 1(,i.e. the end of the period of a being),and the site(volume) of right side is in the interval between 0(,i.e. the beginning of the height of a being,)and 1 (,i.e. the end of the height of a being). 3.Lower side from the (0,0) point to the (1,0) point: The site(volume) of lower side is 0(,i.e. the beginning of the height of a being),and the time of lower side is in the interval between 0(,i.e. the beginning of the period of a being,)and 1 (,i.e. the end of the period of a being). 4.Upper side from the (0,1) point to the (1,1) point: The site(volume) of upper side is 1(,i.e. the end of the height of a being),and the time of upper side is in the interval between 0(,i.e. the beginning of the period of a being,)and 1 (,i.e. the end of the period of a being). Notice that the relative values of site(volume) and time are only in the interval between 0 and 1, but the actual values depend on the height and period of a being. See [Fig. x1] for X_1 square's figure. See [Fig. y1] for Y_1 square's figure.
[Fig. x1]:

[Fig. y1]:

The higher status(ranking) law is that { X_(n+1)=X_n.Y_n. (law A),and Y_(n+1)=Y_n+X_n+ (law B) for n=1 to positive infinity .} (.)'=(+) ,i.e. the complement of (.) is (+), (+)'=(.) ,i.e. the complement of (+) is (.), (X_n)'=(Y_n) ,i.e. the complement of (X_n) is (Y_n), (Y_n)'=(X_n) ,i.e. the complement of (Y_n) is (X_n), De Morgan's law is that { [X_(n+1)]'=[X_n.Y_n.]'= Y_n+X_n+ = Y_(n+1) ,i.e. the complement of [X_(n+1)] is Y_(n+1), and [Y_(n+1)]'=[Y_n+X_n+]'= X_n.Y_n. = X_(n+1) ,i.e. the complement of [Y_(n+1)] is X_(n+1) .} For example, X_2=X_1.Y_1. , Y_2=Y_1+X_1+ , X_3=X_2.Y_2. , Y_3=Y_2+X_2+ , X_4=X_3.Y_3. , Y_4=Y_3+X_3+ , and so on . Apparently the higher status(ranking) law is recursive. The two compositions of a 'pair' must be complementary to each other, and the two statuses(rankings) of the two compositions of a 'pair' must be equal to each other! For example,X_2 and Y_2 are the two compositions of a 'pair', so X_2 and Y_2 must be complementary to each other. The two statuses(rankings) of X_2 and Y_2 must be equal to each other. When the status(ranking) of X_(n+1) is compared with that of X_n, the statuses(rankings) of X_(n+1) and Y_(n+1) are higher,and the statuses(rankings) of X_n and Y_n are lower. The higher status(ranking) is not equal to the lower status(ranking). The higher status(ranking) is higher and better than the lower status(ranking). The lower status(ranking) is lower and worse than the higher status(ranking). I am going to explain the following law: { X_2=X_1.Y_1. } At first,see [Fig. x2],[Fig. x2-2],and [Fig. x2-3] for possible figures of the X_2 square.
[Fig. x2]:

[Fig. x2-2]:

[Fig. x2-3]:

In [Fig. x2],[Fig. x2-2],and [Fig. x2-3] for possible figures of the X_2 square, the black rectangles come surely from the black X_1 square,and the white rectangles come surely from the white Y_1 square. Any possible figure of the X_2 square must satisfy the following 2 laws. 1. In any possible figure of the X_2 square, no matter what time interval the X-coordinates of a black rectangle is in, the Y-coordinates of a black rectangle must occupy 'FULLY AND CONTINUOUSLY' the site(volume) interval between 0 and 1. In any possible figure of the X_2 square, no matter what time interval the X-coordinates of a white rectangle is in, the Y-coordinates of a white rectangle must occupy 'FULLY AND CONTINUOUSLY' the site(volume) interval between 0 and 1. 2. In any possible figure of the X_2 square, the total area of all black rectangles in a square must be 1/2,and the total area of all white rectangles in a square must be 1/2,too. Any square figure satisfying the 2 laws mentioned above is a possible figure of the X_2 square, where { X_2=X_1.Y_1. .} The three statuses(rankings) of [Fig. x2],[Fig. x2-2],and [Fig. x2-3] (for possible figures of the X_2 square) are equal to one another. I am going to explain the following law: { Y_2=Y_1+X_1+ } At first,see [Fig. y2],[Fig. y2-2],and [Fig. y2-3] for possible figures of the Y_2 square.
[Fig. y2]:

[Fig. y2-2]:

[Fig. y2-3]:

In [Fig. y2],[Fig. y2-2],and [Fig. y2-3] for possible figures of Y_2 square, black rectangles come surely from the black X_1 square,and white rectangles come surely from the white Y_1 square. Any possible figure of the Y_2 square must satisfy the following 2 laws. 1. In any possible figure of the Y_2 square, no matter what site(volume) interval the Y-coordinates of a black rectangle is in, the X-coordinates of a black rectangle must occupy 'FULLY AND CONTINUOUSLY' the time interval between 0 and 1. In any possible figure of the Y_2 square, no matter what site(volume) interval the Y-coordinates of a white rectangle is in, the X-coordinates of a white rectangle must occupy 'FULLY AND CONTINUOUSLY' the time interval between 0 and 1. 2. In any possible figure of the Y_2 square, the total area of all black rectangles in a square must be 1/2,and the total area of all white rectangles in a square must be 1/2,too. Any square figure satisfying the 2 laws mentioned above is a possible figure of the Y_2 square, where { Y_2=Y_1+X_1+ .} The three statuses(rankings) of [Fig. y2],[Fig. y2-2],and [Fig. y2-3] (for possible figures of the Y_2 square) are equal to one another. see [Fig. x2] for possible figures of the X_2 square. see [Fig. y2] for possible figures of the Y_2 square.
[Fig. x2]:

[Fig. y2]:

see [Fig. x3] for possible figures of the X_3 square. see [Fig. y3] for possible figures of the Y_3 square.
[Fig. x3]:
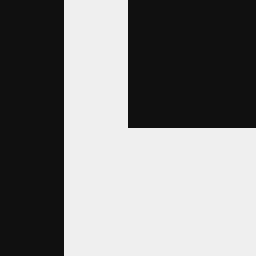
[Fig. y3]:
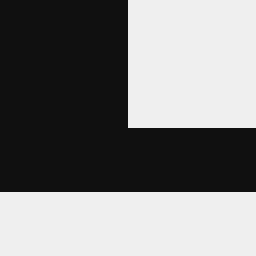
Law of cause-effect: If cause is X_n,effect will be Y_n. If cause is Y_n,effect will be X_n. . The being having the most high status(ranking) is not Buddha! There are many beings who have more high statuses(rankings) than Buddha. For example,'kulaya-raja' has more high status(ranking) than Buddha. Of course,there are also many beings who have more high statuses(rankings) than 'kulaya-raja'. Let Y_1 be anger, then X_1 is love,Y_1 is anger, X_2 is happiness,Y_2 is dharmakaya, X_3 is mahasattva,and Y_3 is buddha. As mentioned above, the total area of all black rectangles in any square must be 1/2,and the total area of all white rectangles in any square must be 1/2,too. But it seems that the total area of all white rectangles in the fully black X_1 square is zero instead of 1/2, the total area of all black rectangles in the fully black X_1 square is one instead of 1/2, the total area of all black rectangles in the fully white Y_1 square is zero instead of 1/2,and the total area of all white rectangles in the fully white Y_1 square is one instead of 1/2. I am going to solve the 'single color' problem mentioned above in the following. In fact, [X_1]=[X_0].[Y_0]. , [Y_1]=[Y_0]+[X_0]+ , [X_0]=[X_-1].[Y_-1]. , [Y_0]=[Y_-1]+[X_-1]+ , [X_-1]=[X_-2].[Y_-2]. , [Y_-1]=[Y_-2]+[X_-2]+ , etc. , for index = 1,0,-1, etc. , to negative infinity. It seems that both black rectangles and white rectangles disappear from the squares of X_index and Y_index , for index = 0,-1, etc. , to negative infinity. In order to solve the 'single color' problem,we must let (,let's say,) X_-100 be a fully (,let's say,) green square without red rectangle,and let (,let's say,) Y_-100 be a fully (,let's say,) red square without green rectangle. Both green rectangles and red rectangles will appear surely in the squares of X_index and Y_index , for index = 1,0,-1, etc. , to -99. Although the black X_1 square has only a color, there are both green rectangles and red rectangles in the X_1 square. Although the white Y_1 square has only a color, there are both green rectangles and red rectangles in the Y_1 square. Both green rectangles and red rectangles can solve the 'single color' problem in the squares of black X_1 and white Y_1.
p.s. please go to: https://mavang2.tripod.com/square/correct.txt